ITEM!: In Word Ways #1.2, “Renaming the Numbers,” Rudolf Ondrejka built on Dmitri Borgmann’s earlier piece to suggest a naming scheme for really, really big numbers. (Others would try this too in later issues.) Well, really two naming schemes, conforming with the then-different numbering systems used in the U.S. and the U.K.:
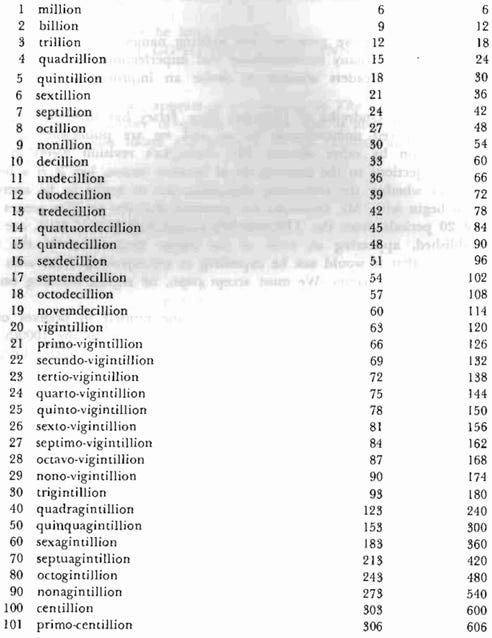
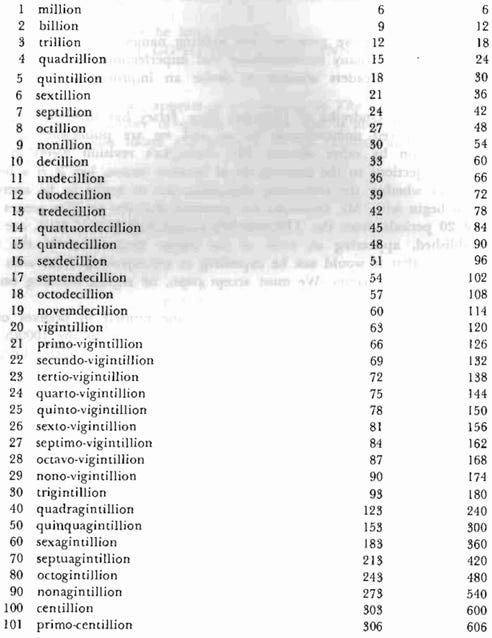
Nowadays, the U.S. system has become standard. Using this scheme, a googol (1 followed by 100 zeroes) would be ten secundo-trigintillion. A googolplex, 1 followed by a googol of zeroes, would still be out of reach. The article mentions googolplex, as well as a few even larger numbers: Skewes’s number, the mega, the megiston, and the moser. How big are these?
There are infinitely many Skewes’s numbers, but the smallest known one is approximated as 1010^10^34. That beats the googolplex’s 1010^10^2. Already, we’re leaving the observable universe: there aren’t enough atoms anywhere to write all the zeroes you’d need to represent these figures. And the other numbers make this one look dinky.
A mega is…well. Start with 256256^256^(256+1). That’s a chain of three exponents, right? If you extend that power tower to 256 exponents, keeping all of them at “256” except for the final one, which is 256+1 (257), then you’ve got a mega.
A megiston is what you get when you take the above paragraph and swap all the 256s out for 1010^10^10s. (The 257 gets swapped out for 1010^10^10 + 1.)
A moser is 2 multiplied by itself a “mega” number of times.
A few numbers dwarf even these and require more advanced math to even explain. Graham’s number once held the record for the “largest number used in a serious mathematical proof,” but since then, at least two larger ones have emerged: TREE(3) and Rayo’s number. The latter is possibly the largest named finite number. More about these some other time. An avid mathematician, Rudolf Ondrejka left a list of “top ten primes” (as then known) as part of his legacy.
…
ITEM!: One of my inspirations for getting into Trump’s language on Tuesday was a piece from Word Ways #1.2 by Rudolph W. Castown called “Imitating Ho Chi Minh.” (A lot of Rudolfs in that issue!) Back in 1968, America was at war with North Vietnam (you might’ve heard something about that), and the ailing Minh was still North Vietnam’s head of state.
Though support for the war was fast eroding in America, that was still a provocative title.
But Castown’s angle seemed apolitical. As a younger man, Minh had composed a series of Chinese poems while incarcerated…titled “Word Play”! The gimmick involved altering Chinese characters: Take away the bamboo top from the sign for prison. That gives you dragon… When the prison doors are opened, the real dragon will fly out.
Little is known about Rudolph W. Castown today, but he was a founding member of the Futurians, an early science-fiction club that promoted communism in the 1930s, when such ideas were fashionable. What did Castown think of communist Minh in 1968? Aside from prefacing his work with “Famous—or infamous—statesmen are not above indulging in word games,” which seems like a condemnation but isn’t quite…he kept that to himself.
…
ITEM!: A while ago, Randall Munroe’s xkcd published this bit of “space typography” (astrotype?):
One can extend this exercise to placements for Saturn, Uranus, and Neptune. I’ve taken a stab at it below:
Optimistic aliens measure space typographically, but some planets have a greater distance between them than others, and that fact can cause a formerly easy mnemonic to grow as bloated as the padded, pompous, ponderous sentences of the average teenage essayist.



As a noted side show performer said about weighing 600 pounds -- large is all relative,
When I went to my ATM and saw the nunber 257, I thought that was a large number.
To Donald Trump $257 does not exist. For mathemjaticians it could be a small prime
number, but to a child it could be a huge prime number. On the other hand, my I. Q.,
would never be a large number.